Zhaoyang Zhang, Yuan Feng, Feng Li, Sergei Koniakhin, Changbiao Li, Fu Liu, Yanpeng Zhang, Min Xiao, Guillaume Malpuech, and Dmitry Solnyshkov Angular-Dependent Klein Tunneling in Photonic Graphene Phys. Rev. Lett. 129, 233901 https://doi.org/10.1103/PhysRevLett.129.233901
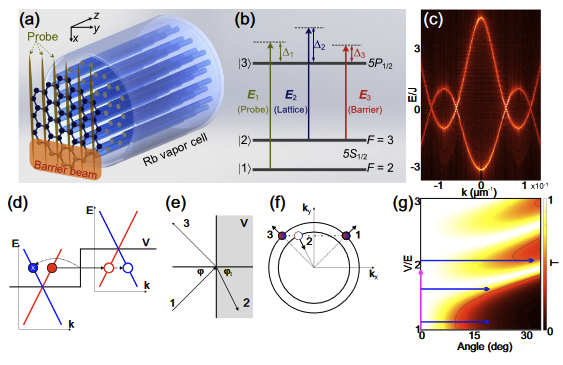
The Klein paradox consists in the perfect tunneling of relativistic particles through high potential barriers. It is responsible for the exceptional conductive properties of graphene. While in theory the perfect tunneling holds only for normal incidence, so far the angular dependence of the Klein tunneling and its strong variation with the barrier height were not measured experimentally. In this Letter, we capitalize on the versatility of atomic vapor cells with paraxial beam propagation and index patterning by electromagnetically induced transparency. We report the first experimental observation of perfect Klein transmission in a 2D photonic system (photonic graphene) at normal incidence and measure the angular dependence. Counterintuitively, but in agreement with the Dirac equation, we observe that the decay of the Klein transmission versus angle is suppressed by increasing the barrier height, a key result for the conductivity of graphene and its analogs.